How positioning works
This article is part of the Pozyx Academy and is the first in a series of 5 articles that explain how the Pozyx Technology works and what to account for when installing the Pozyx System.
- How positioning works
- How ultra-wideband works
- Positioning protocols explained
- Ultra-wideband and obstacles
- Where to place the anchors
Depending on the technology, positioning can be done in multiple ways. But in general, only two things are needed to perform localization: measurements and reference points.
Measurements
In a way, almost anything you can measure depends on the position it has and could be used for positioning. You could for example use the temperature to determine in which continent you are, but that would not be very accurate. For more accurate results, it makes more sense to measure the distance or the angle to some point.
Reference points
It is only possible to describe a position relative to a reference point. Reference points could be your home, a lighthouse, the north star or satellites.
Ultra-wideband technology uses anchors as reference point. To describe a two-dimensional coordinate system, three anchors are sufficient. To position in three dimensions, four anchors are required.
Trilateration
The most commonly used method of positioning uses basic geometry to calculate the position. By measuring the distance from the asset to the reference point, it is possible to determine that there is a circle of that radius around the reference point. If we do this with three different reference points, we see that the position of the asset is uniquely determined by the intersection of the three circles. This method is called trilateration (or multilateration if more than 3 anchors are used).
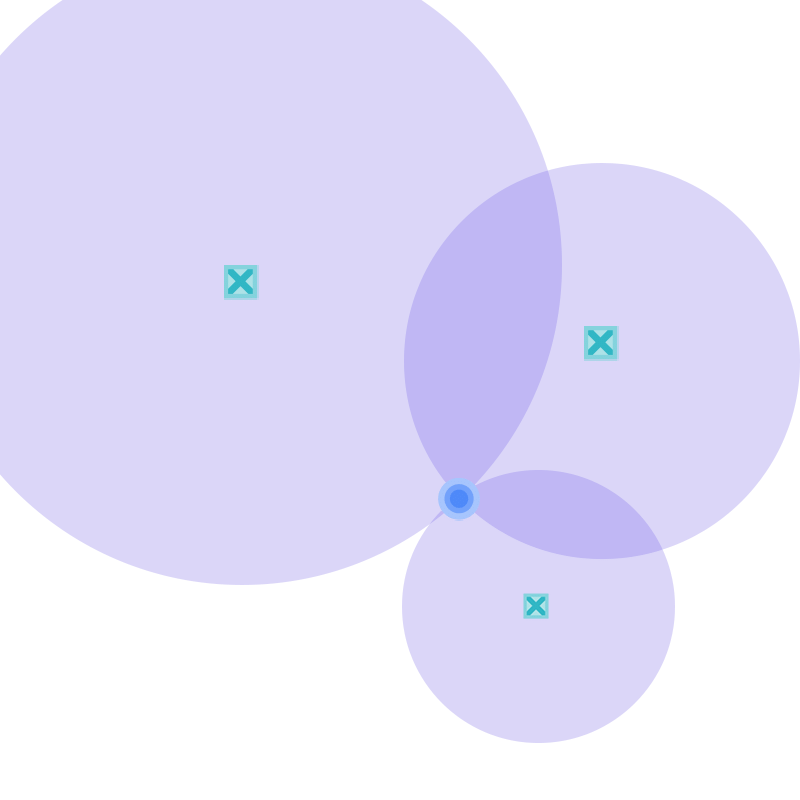
The difficulty of this approach lies in the fact that the measurements are not perfect. There will always be some noise on the measurements and because of this, the circles will not intersect at exactly one point. To circumvent this issue, the Pozyx System pinpoints the intersection that is closest for all circles to obtain exact location data.
This is generally how positioning works. By measuring the distance between the asset and its reference points, we can calculate the location of the asset.
Read the next article on ultra-wideband technology to learn more about Pozyx’s technology.





